Areas
Disease evolution and the tragedy of the commons
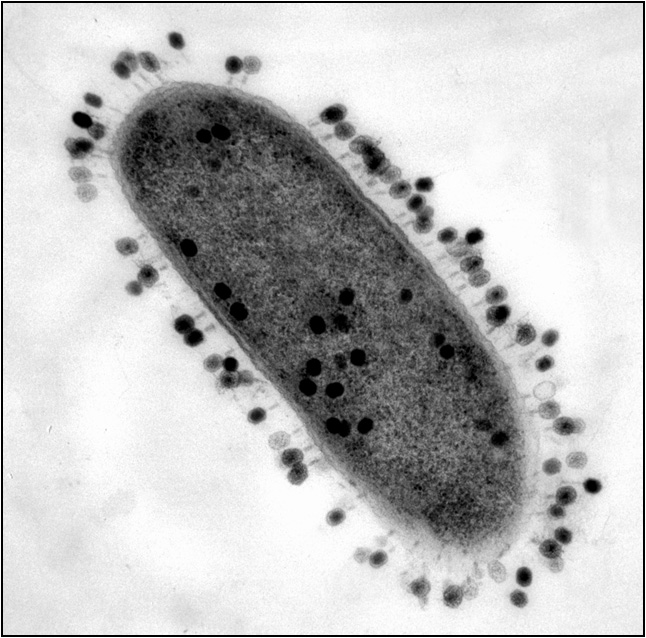
Figure 1: A micrograph of an E. coli cell being infected by several T4 particles (TEM courtesy of J. Wertz). The virus binds to the surface of the bacterium and injects its DNA. After production of new phage particles, the bacterium lyses and the viral progeny are released.
We have developed a microbial host-pathogen system to study disease dynamics in metapopulations. The host is the bacterium Escherichia coli and the pathogen is a virus, phage T4, which infects and kills the bacterium (Figure 1). We embedded populations of the bacterium and phage in 96-well microtiter plates (Figure 2). Each media-filled well was a subpopulation nested within the larger collection. We used a high-throughput liquid-handling robot (Figure 3) to transfer small portions of one subpopulation from its natal subpopulation to a new subpopulation. Thus, the robot functioned as a ‘migration machine’ and we could program it to create any set of ‘transportation routes’ between subpopulations. In this way, we could control the structure of the network of subpopulations (i.e., how hosts and their pathogens contact one another).
Using this robot, we have experimentally manipulated the pattern of migration between subpopulations. In one treatment (Restricted), migration events only occurred between neighboring wells. That is, migrants from one subpopulation only moved to a subpopulation that is directly north, east, south or west. In another treatment (Unrestricted), migration events took place between any two subpopulations in the entire collection.
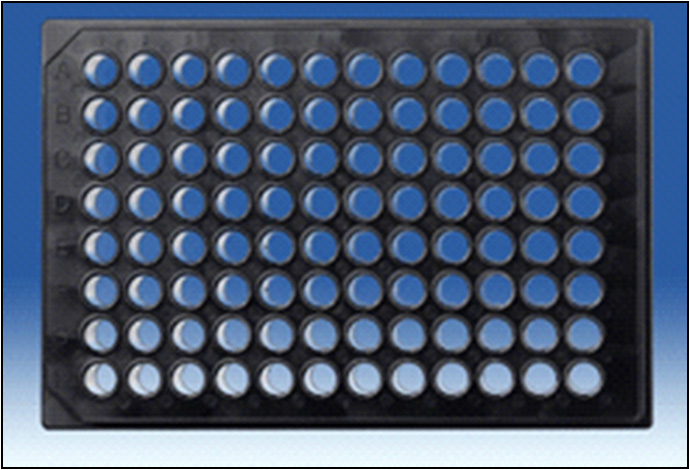
Figure 2: A 96-well microtiter plate. Each well constitutes a microbial subpopulation and is filled with bacteria, virus or both. Migrations between wells occur according to some specified experimental treatment.
After nearly 70 viral generations, we measured the productivity and competitive ability of pathogens from different migration treatments. Regardless of the initial multiplicity of infection (MOI, or ratio of phage to bacteria), phage from the Unrestricted treatment were significantly less productive than phage from the Restricted treatment. However, phage from the Unrestricted treatment were significantly more competitive than phage from the Restricted treatment (in competition with a common “marked” phage strain). This is consistent with a tradeoff between competitive ability and productivity and indeed we found negative correlations between these phage phenotypes.
These findings suggest the possibility of a ‘tragedy of the commons’, a scenario that arises when multiple users exploit the same resource. As unrestrained users displace restrained competitors, the resource (the ‘commons’) is over-exploited, lowering overall user productivity (the ‘tragedy’). The bacteria in each well are a common resource for resident phage. Prudent use of this resource can lead to higher productivity of the phage (e.g., if phage lengthen the time spent within a host before lysing it, then uninfected hosts may continue to replicate ensuring extended resource access). Rapacious phage can out-compete their prudent cousins by limiting future access to the host through their own rapid host consumption. The ‘tragedy of the commons’ is that rapacious types lower overall productivity as they displace more restrained competitors.

Figure 3: One of the high-throughput liquid handling robots used in the experiments. The robot arm sends down pipettes to transfer small portions of one well to another well within a microtiter plate on its platform (i.e., the robot executes migrations between microbial subpopulations).
Different patterns of migration favor different resolutions of this tragedy. Within either spatially structured treatment, any rapacious phage mutant should out-compete its ancestor and lower overall productivity of the well. Given periodic dilution however, less productive phage subpopulations run a higher risk of extinction. The success of rapacious phage therefore depends on sufficient access to fresh hosts to compensate for lower productivity. Such access is ensured by unlimited migration in the Unrestricted treatment. By contrast, spatially restricted migration reduces the probability that phage reach fresh hosts, rendering rapacious subpopulations more prone to extinction through dilution. Consequently, the phage in the Restricted treatment remain relatively prudent by default.
What makes phage evolving in the Unrestricted treatment more rapacious? We have found that phage from the Unrestricted treatment are more infective and more virulent than phage from the Restricted treatment. By quickly entering and exiting hosts, the infective/virulent virus out-competes other strains, but it is less productive due to quick overexploitation of the hosts. We have evidence that single frameshift mutations in gene rI (coding for the phage antiholin) are responsible for shifts in virulence. Currently, we are exploring a new set of network topologies and alternative host-pathogen systems using the high-throughput robot, in order to probe how and when the structure of the network favors pathogen rapacity or virulence.
Readings:
The ecology and evolution of a microbial game of rock-paper-scissors
Colicinogenic strains of the bacterium Escherichia coli are potent niche constructors. These strains excrete a plasmid-encoded toxin (colicin) which kills susceptible bacterial cells. In natural communities, a colicin-producing strain (immune to the action of its own toxin) often coexists along with strains sensitive to the toxin and other strains resistant to the toxin (strains that can grow in the presence of the toxin, but do not produce the toxin). In some cases, the growth rate of the sensitive strain (S) will exceed that of the resistant strain (R), which exceeds that of the colicinogenic strain (C). This is because there are costs to being resistant and even greater costs to producing the toxin. However, because C produces a toxin that kills S, we have the following pairwise interactions: C kills S, S outgrows R, R outgrows C. That is, we have a rock-paper-scissors relationship.
We have constructed lattice-based computer simulations to explore the role of spatial structure in the maintenance of strain diversity within this community. We found that when cell birth and interaction occurred locally, that all three types persisted indefinitely; whereas diversity was rapidly lost when the community was “mixed” (i.e., when birth and interactions occur over large distances). When ecological processes were localized, “clumps” of the three types formed and these clumps chased one another around the lattice forming a fluid mosaic in which diversity was maintained. When the community was mixed, the entire population was exposed to the niche construction effect of C (i.e., the excreted toxin); consequently, S was driven to extinction and then R outcompeted C.
We then tested these theoretical predictions in the laboratory with three strains of E. coli (C, S, and R) satisfying the non-transitivity. Our “structured” environment was the surface of an agar plate (Figure 5). We serially transferred our population from a nutrient-exhausted plate to a fresh plate by stamping the exhausted plate on a sterile taught velveteen cloth and then stamping the fresh plate on the cloth; this procedure maintains the spatial relationships in the community across transfers. Our “mixed” environment was a shaken flask with liquid broth inside. In line with our theoretical predictions, we found that our structured communities maintained their diversity for the entire run (nearly 70 generations), whereas the community in the flask was quickly dominated by one type (R).
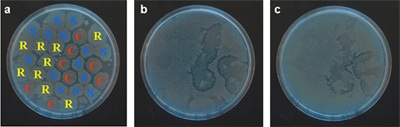
Figure 5: A biofilm rock-paper-scissors community on the surface of a Petri dish. The community is initialized by placing droplets of pure cultures of the colicin-producer (C), sensitive strain (S) and resistant strain (R) randomly in a hexagonal lattice pattern. The community is shown after 3 days (a), 5 days (b) and 7 days (c), where replica plating transfers took place daily. Because producers are poor growers, they form patches of lower density, which allows boundaries between producer patches and patches of the other strains to be followed over time. Careful inspection of these boundaries reveals that C chases S, and R chases C (as in Figure 4).
In our first experiment, we found resistant mutants that arose de novo, which suggested that evolutionary phenomena should be considered within this system. Using a lattice-based simulation approach, we allowed strain properties to evolve. Interestingly, in spatially-structured communities, we find that strains do not evolve to maximize their competitive ability: They do not reduce their probability of death to a minimum nor increase their toxicity to a maximum. We show that non-transitivity and spatial structure are both necessary for the evolution of restraint. In a non-transitive threesome, improving the growth rate of one strain can lead to a lowering of its local density or even its eventual local extinction! This is because by chasing the enemy of your enemy faster, you are helping liberate your enemy. In essence, we find evolutionary resonance with the aphorism “the enemy of my enemy is my friend.” We are currently testing the evolution of restraint in this system using experimental metapopulations and high-throughput robotics (see previous section).
Finally, in collaboration with Peg Riley and Osnat Gillor, we are currently doing theoretical and experimental work on the eco-evolutionary role of cross-induction (the colicin of one strain inducing production of a different species of colicin in another strain) or auto-induction (the colicin of one strain inducing colicin production in the same strain).
Readings:
The evolution of plant flammability and genetic niche-hiking

Figure 6: A pine stand outside of Yosemite after an intense fire. Despite the destruction, a flush of new tree growth is detected.
From the Californian chaparral to the Australian eucalypt, plants possess traits that allow them to persist in fire-prone ecosystems (i.e., thick bark, resprouting capability, serotinous cones, etc.; see Fig. 6). Perhaps surprisingly, some of these same plants possess traits that actually enhance their flammability (e.g., retention of dead branches, possession of volatile secondary compounds, sclerophylous leaf structure, etc.). Forty years ago, Robert Mutch suggested that plants that are already tolerant of fire might evolve to be more flammable in order to fend off superior competing plant species that were fire-sensitive.
In collaboration with Dylan Schwilk, we have investigated the evolution of flammable traits using a population genetic model. We were interested not only in the flammable traits themselves, but also the effect that such traits would have on the evolution of other plant traits, such as plant growth in a fire-cleared gap. We used a two-locus model, with alleles at one locus (the flammability locus) influencing the flammable character of the plant, while alleles at the other locus (the disturbance locus) influenced the success of the plant as it grew either in a fire-cleared gap or through a stand of conspecifics. The niche construction effect was quantified as a parameter that represented the impact that flammable plants had on the fraction of the population that burned.
We found that stable polymorphisms at the flammability locus were possible—that is, the maintenance of both flammable and less flammable plant types. We found potential support for the converse of Mutch’s hypothesis: if plants possess traits that enhance their flammability, plants may evolve fire-adapted traits. Specifically, if there is any reason why plants become more flammable (for instance, volatile compounds deter herbivores), selection engendered by the flammable character would favor traits that allowed the plant to deal with the enhanced presence of fire (for instance, ability to grow well in a fire-cleared gap). Within the context of our model, the evolutionary trajectory at the disturbance locus can depend on the frequencies of alleles at the flammability locus. The basic idea is that the plant acts as a co-author in its own evolution through the effect it has on its environment. Much of our work on this model is centered on deducing criteria for the stability of a genotype or combinations of genotypes to invasion by other genotypes. As expected, both the rate of recombination between loci and the genotypic fitnesses find their way into these stability criteria. However, predictions of evolution at the disturbance locus based on genotypic fitnesses alone can fail. This shortcoming is due to neglect of the effect that the plant has on its own selective environment.
In our population genetic model, flammable types only evolve if there is a direct advantage to being flammable. However, we used a stochastic cellular automata approach to demonstrate that flammable traits can increase in frequency even without direct fitness benefits. In this simulation work, flammable types “ignite” and burn their neighbors to produce local gaps (the constructed niche) in which their offspring are able to continually track a changing environment. This environmental tracking occurs at (fitness) loci unlinked to the flammability locus. However, an association can develop between current good combinations of fitness alleles and the flammability allele if mating, dispersal and niche construction all occur locally (in such a spatially structured system, the offspring are likely to experience the niches their parents construct). In analogy to genetic hitchhiking (in which a deleterious allele can invade due to a genetic linkage) we call this process “genetic niche-hiking,” because the association results from localized niche construction. Genetic niche-hiking may also be applicable to the evolution of other (seemingly counterintuitive) traits in spatially structured ecological systems such as plant disease susceptibility and tree characteristics associated with gap formation.
Readings:
The evolution of cognition
Why do different organisms process information in their worlds so differently? Why do some animals possess remarkable cognitive flexibility, while others display more rigid responses to their environments? To explore these issues, we have constructed mathematical and simulation-based approaches to study cognition. In these models, an organism samples behaviors from a repertoire in response to a stimulus. The behavior that yields the highest payoff can be recorded into memory and reused if the stimulus is experienced again. The value of memory will depend on the reliability of the stored information; that is, how the payoffs for each behavior change over time. The organism may also choose the stimulus to which it responds. This choice will depend on the information in the organism’s memory as well as the number of stimuli that the organism can sense. The number of behaviors sampled, the memory length, and the number of stimuli observed are variables in the model that define the learning strategy. When the stimuli are homogeneous in their reliability, we find only a single globally optimal learning strategy. However, when there is heterogeneity in stimulus reliability, multiple locally optimal learning strategies may exist. In some cases these strategies correspond to specialists and generalists. More recently, we have focused on memory as a potentially dynamic component of cognition. Using a dynamic programming approach, we have focused on how optimal memory length changes as a function of age and condition of the learning animal as well as stimulus reliability. As before, greater stimulus reliability favors longer memories across lifetimes. However, memory length can change within a lifetime according to the state the individual is in, and the metabolic costs of living (we predict that an individual in a poor state, with a high cost of living should have long memories, while an individual in a good state, with a low cost of living, should have very short memories). Also, we find that there are circumstances where it is optimal for memory length to decline towards the end of the lifetime. Because our model does not take into account the degradation of the neural mechanisms, our result implies that memory loss with age might actually be adaptive.
Within the models described above, stimulus reliability is a static parameter. However, learning organisms potentially alter the form and abundance of their stimuli by the behaviors they choose in response to these stimuli. For instance, butterflies drain nectar reserves from flowers as they learn nectar extraction technique and birds consume butterflies as they learn to identify and capture them. In these cases, the food resources (or stimuli) are changed by the action of the learning organism. Consequently, stimulus reliability will depend on the manner in which learners interact with stimuli. Such biotic effects will change not only the value of specific behaviors in the future, but also the value of entire learning strategies. We have built learning models that simultaneously track the stimulus population and the learning population. These models are coevolutionary – the learning organism’s strategy is modeled using a linear operator framework (common in mathematical psychology) such that the rules of learning and the initial behavioral biases (e.g., preferences for certain stimuli and proclivities to react in certain ways) can evolve in response to the evolving stimulus population. When the stimulus and responding agent have common interests (positive niche construction) rapid learning and fixed behavioral distributions evolve. When the stimulus and responding agent have divergent interests (negative niche construction) slower learning and flexible behavioral distributions evolve. Thus, we find that the form of niche construction can have profound effects on the evolution of cognition.
Readings:
The levels of selection

Figure 7: In this famous cartoon by W.E. Hill, both a young woman and older woman are displayed.
In collaboration with Peter Godfrey-Smith, we have written about different mathematical perspectives in the levels of selection debate. Natural selection in populations with group structure can generally be described from different perspectives (just like the famous cartoon from W. E. Hill, see Figure 7). We have shown that the standard multi-level perspective and individualist perspective are mathematically interchangeable. Further, we suggest that there are heuristic advantages to keeping both perspectives in mind when approaching selection in structured populations. Thus, we advocate a kind of “gestalt-switching” pluralism. This work has led to a reconsideration of the definition of “altruism” as well as some statistical descriptions of evolutionary change that naturally translate to selection in group-structured populations (e.g., the famous Price equation). Currently, we are working on rigorous tests to determine when one perspective better captures the causal story of selection in particular case studies. This work places us in an interesting, but murky, philosophical realm, in which the nature of causality itself becomes an issue.
Readings: